Piececlopedia: Unicorn (3D)

Historical notes

The Unicorn was invented for the 3D Chess variant Raumschach by Ferdinand Maack in 1907. In that game, the Rook moves through one dimension at a time, the Bishop moves through two dimensions at a time, and the Unicorn moves through three dimensions at a time. Because this is a thoroughly 3D piece, it is immobile on a 2D board, which has stopped it from being used in 2D games, though the name has been used in other games for pieces with different powers of movement.

Movement

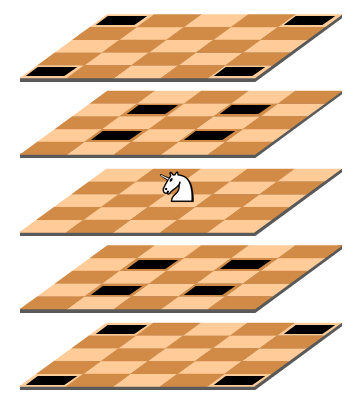
Like the Rook and Bishop, the Unicorn is a rider that moves one space at a time along a straight line. Each step of a Unicorn's move takes it one space away in all three dimensions. It may continue moving in exactly the same way until it stops on an empty space or it reaches an occupied space. If the space is occupied by an opponent's piece, it may capture it by displacement. Otherwise, it must stop before the piece in its way.

Notes

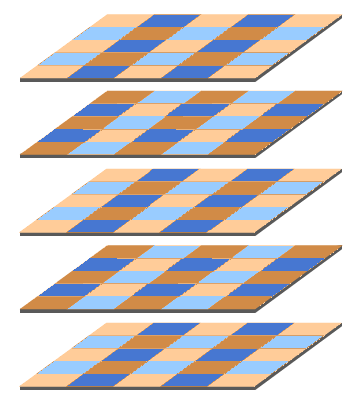
In two moves, assuming it isn't blocked, a Unicorn may reach any space that a 3D Dabbabah could reach, which is to say any space two spaces away in a single dimension. So, on any given plane, it may eventually reach all the spaces a Dabbabah could reach. Unlike the Dabbabah, though, it can also move to an adjacent plane. This gives it the ability to reach the same area as two different Dabbabahs placed on different planes could reach. Although a Dabbabah can reach only one quarter of the spaces on a Chess board, its inability to move to an adjacent plane causes it to cover only one eighth of the spaces on an 8x8x8 board. Thanks to not having this inability, the Unicorn can reach one fourth of the spaces on an 8x8x8 board. So it would take four Unicorns to cover the full board. On the 5x5x5 board depicted here, it would still take four Unicorns to cover every space, but there would be differences in how many spaces each Unicorn can reach. Despite this, Raumschach gives each player only two Unicorns.

Piece Graphics